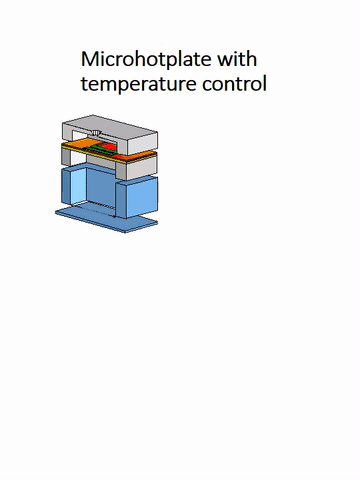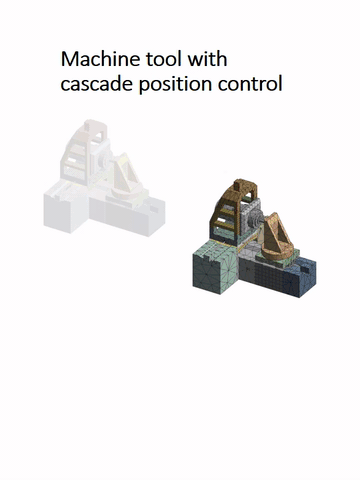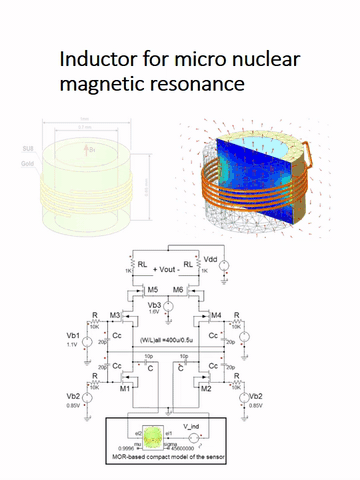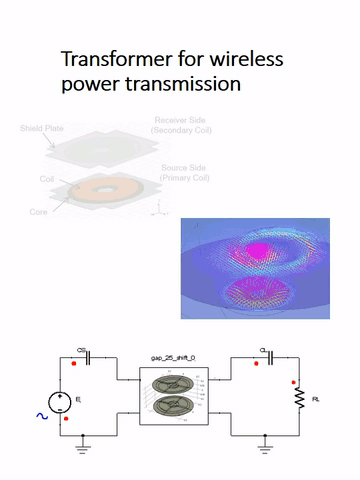
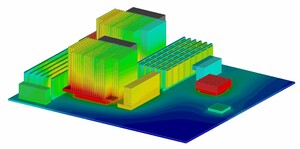
Modern mechatronic components operate based on principles of different physical domains. In addition, they often feature complex geometries. Modelling such components as a continuous systems, i.e. with partial differential equations, is highly complex and requires time-consuming numerical solutions. The finite element method is capable of these computations. As soon as the mechatronic components are to be simulated together with the associated control system, housing or other components, the models have to become more efficient.

Mathematical methods of model order reduction offer one approach to create compact but still accurate models. Reduced models drastically increase the computational efficiency of system simulations and design optimizations.

Steinbeis-Transferzentrum

Before a technical system is built, its behavior can be examined in computer simulations and using digital twins. The Steinbeis Transfer Center Finite Element Simulation, Model Order Reduction and Design Optimization is happy to offer you this service. We work on mechanical, thermal, electromagnetic and fluidic problems. Contact us to discuss and work on your modeling and simulation needs.